RESOURCES > EIS
> DIFFUSION
 Whenever you look at a Complex-Plane Impedance Plot (
Nyquist or Cole-Cole plot) and see a 45° line, or fit data to an equivalent circuit and find a
Constant Phase
Element (CPE) with an n-value close to 0.5, you should consider
diffusion as a possible explanation. Whenever you look at a Complex-Plane Impedance Plot (
Nyquist or Cole-Cole plot) and see a 45° line, or fit data to an equivalent circuit and find a
Constant Phase
Element (CPE) with an n-value close to 0.5, you should consider
diffusion as a possible explanation.

MORE
 Diffusion Circuit Elements - Warburg
Diffusion Circuit Elements - Warburg
The most common diffusion circuit is the so-called "Warburg"
diffusion element, but it is not the only one! A Warburg impedance element
can be used to model semi-infinite linear diffusion, that is, unrestricted
diffusion to a large planar electrode. This is the simplest diffusion
situation because it is only the linear distance from the electrode that matters.
The Warburg impedance is an example of a
constant phase element for which the phase angle is a constant 45� and
independent of frequency. The magnitude of the Warburg impedance is
inversely proportional to the square root of the frequency ( 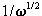 )
as you would expect for a CPE with an n-value of 0.5. The
Warburg is unique among CPE's because the real and imaginary components are
equal at all frequencies and both depend upon )
as you would expect for a CPE with an n-value of 0.5. The
Warburg is unique among CPE's because the real and imaginary components are
equal at all frequencies and both depend upon 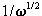 . .
 Warburg Equations
Warburg Equations
The equation for a Warburg's impedance ( Zw
) is a
simple one, but the equation for the Warburg constant (  ) is a bit more complicated and will be discussed on another
page.
) is a bit more complicated and will be discussed on another
page.
|